

Can you figure out errors in the second and fourth quadrants? The angle variations are plotted showing by comparison that starting deLaHire polar line is inclined more than (or equals to at extreme axes) the Central polar coordinate always. In either case polar angles $\theta = 0$ and $\theta= \pi/2$ reach to the same points at the ends of major and minor axes respectively. To more clearly distinguish between them we should note there are two different $\theta$ s, viz $\theta_ \tag 2 $$ The Ellipse parametrization is done differently. To extend this to a circle with any radius, simply scale this by the radius, so the arc length at θ at the center of a circle is r*π.What you at first proposed as ellipse looks like: In other words, radians express the arc length formed by some angle θ at the origin of the unit circle. A few other resources that discuss radians, including some interesting history are:Ī radian is a way to express parts of a circle or divide up a circle into parts by measuring the length around the unit circle between two lines by extending two line segments from the center to the edge of the circle to form an angle at the center. This is here to provide a holistic approach to the topic.
This gives two values for use in the Pythagorean Theorem withĪ 2 + b 2 = c 2 Radians Because the hypotenuse of the triangle goes from the origin to the edge of the circle, it is a radius of the unit circle and thus 1 unit in length. This now makes a right triangle with a base of ½ because it is the perpendicular bisector of a line segment that is 1 unit in length.
Connect the point of intersection on the circle with the origin with a straight line segment. Then construct the perpendicular bisector of the x axis between the origin and the point (1,0) and a line segment that goes from the midpoint on the x axis through the intersection point of the perpendicular bisector and the unit circle. Completing a 360° rotation around the unit circle by adding 90° one more time puts the radius back at the point (1,0), the start point. I tried a similar strategy by going from Cartesian to polar. Ive found a lecture handout (pdf) in which the equation is derived for a circle that is off-centre by exactly its radius. Im trying to come up with the polar equation for an off-centre circle that has a certain radius.

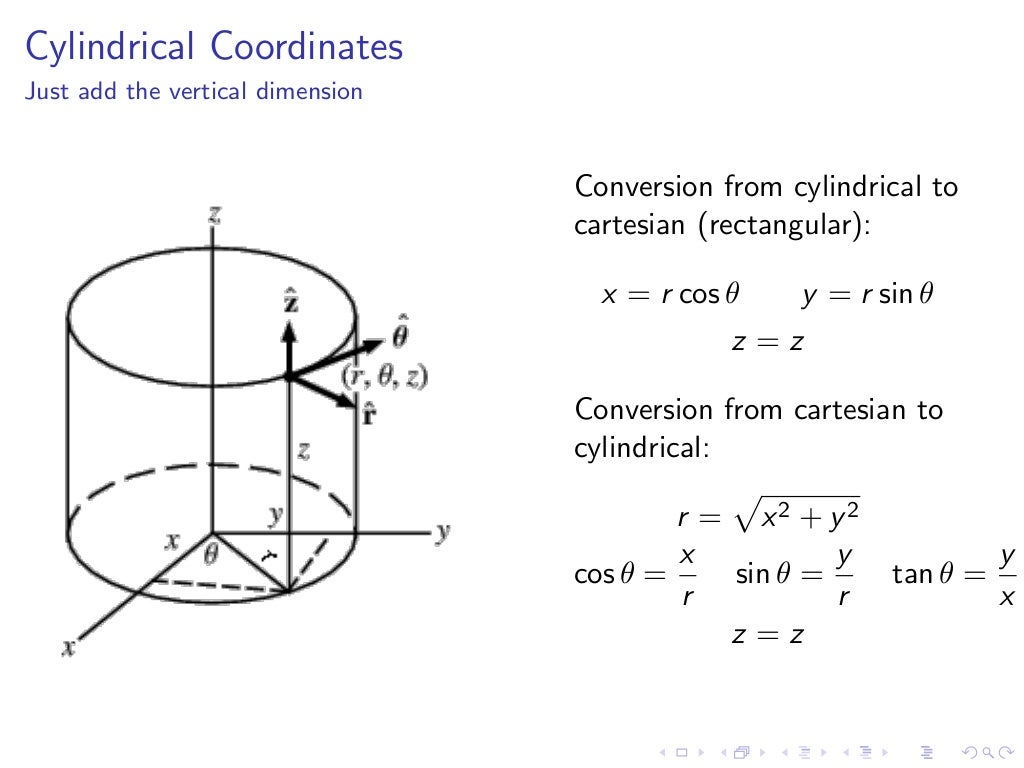
Repeating this addition of 90° from the 180° rotated radius touching (-1,0) would be a total rotation of 270° and would rotate the unit radius down to touch the point (0,-1). Off-centre circle given a radius in polar coordinates. By definition of the Cartesian axes, the angle formed by the 1 unit long radius at this point and the positive x-axis is a 90° angle, the same angle separating the y-axis and x-axis.Īdding 90° to this angle between the unit radius and the positive x-axis at the point (0,1), the radius would then have rotated 180° from its starting position on the positive x-axis and will intersect the edge of the circle at (-1,0), so this point is reached by a 180° rotation. Then consider (0,1), which lies on the y-axis. At this point, there has been no rotation of the radius around the circle yet. From the common notion of a unit circle, let this start point be defined by the radius forming a 0° angle with the positive x axis, which gives the first of these four points, (1,0). To do this, it is necessary to construct the unit circle, which can be defined as a circle with radius 1 centered at the origin, (0,0).Ĭonstruct this circle and label each point where the circle intersects the axes, giving (1,0), (0,1), (-1,0), (0,-1).ĭefine a start point for the radius. To find where Polar Coordinates come from and why they matter, it is important to start with a thorough understanding of the unit circle and how this leads to definitions of sine and cosine. Here are a few that are examples of the majority of information available online about the unit circle: There are tons of websites that talk about the unit circle, but few take the time to derive it and really dig into it. Khan Academy is a 501(c)(3) nonprofit organization. 4 Polar Coordinates and the Complex Plane Our mission is to provide a free, world-class education to anyone, anywhere.3 Relating Graphs Between the Cartesian and Polar Planes.2.1 From Cartesian To Polar Coordinates.2 Polar coordinates and Defining the Polar Coordinate Axes.1.1 Where Trigonometric Functions come from on the Unit Circle.


 0 kommentar(er)
0 kommentar(er)
